Tram of Thought: Comic strip about psychology, philosophy, society, engineering, and just life in all its gory glory

Spacetime

On the first image, a Triangle-person is drawn sitting on a boat looking into the distance. A vector is shown depicting the boat traveling upwards at a velocity of c. Two axes are also drawn, one point up - "time" and one pointing right - "space". The label says: "We are all lonely travelers through space-time" Second image shows the same situation, except the Triangle-person is looking through a looking-glass. The label says "Always moving at the speed of 1 lightsecond per second (c=299,792,458 m/s)". On the third image, there is two boats. The Triangle-person boat is still moving upwards, while the Circle-person boat is moving mostly away, and a bit up, also at a velocity of c. Triangle-person tries to reach towards the Circle-person but is too far. Label says: "Looking at others spending their precious velocity to travel also in space." Fourth image shows the Circle-person boat tackling back towards the Triangle, but it's clear from their vector they will not be able to catch up. Label says: "And they can never catch back to us". Fifth image shows Circle-person meeting up with a ghost depcition of Triangle person that is shown below the solid Triangle-person's boat. Label says "Or at least not the same us." Sixth image shows Triangle-person on their boat, symbolically reaching for a star. Label says: "We are all lonely travelers through space-time."

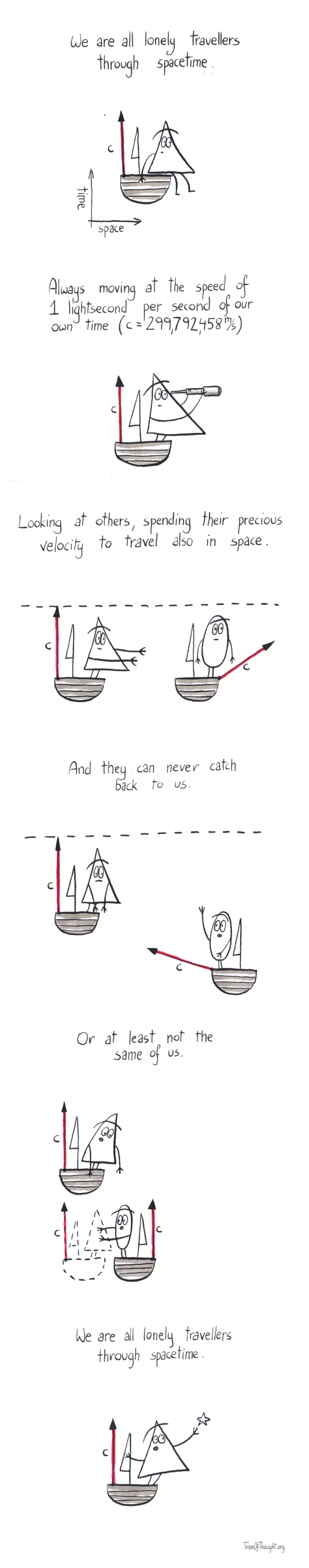
On the first image, a Triangle-person is drawn sitting on a boat looking into the distance. A vector is shown depicting the boat traveling upwards at a velocity of c. Two axes are also drawn, one point up - “time” and one pointing right - “space”. The label says: “We are all lonely travelers through space-time”

Second image shows the same situation, except the Triangle-person is looking through a looking-glass. The label says “Always moving at the speed of 1 lightsecond per second (c=299,792,458 m/s)”.

On the third image, there is two boats. The Triangle-person boat is still moving upwards, while the Circle-person boat is moving mostly away, and a bit up, also at a velocity of c. Triangle-person tries to reach towards the Circle-person but is too far. Label says: “Looking at others spending their precious velocity to travel also in space.”

Fourth image shows the Circle-person boat tackling back towards the Triangle, but it’s clear from their vector they will not be able to catch up. Label says: “And they can never catch back to us”.

Fifth image shows Circle-person meeting up with a ghost depcition of Triangle person that is shown below the solid Triangle-person’s boat. Label says “Or at least not the same us.”

Sixth image shows Triangle-person on their boat, symbolically reaching for a star. Label says: “We are all lonely travelers through space-time.”

According to Einstein’s theory of relativity we all move through spacetime (3 dimensions of space + 1 dimension of time) at exactly the speed of light (c), or “1 lightsecond per second of our own time.” This isn’t optional or variable - it’s a fundamental constant of our universe.

Think of it this way: you have a “budget” of movement equal to the speed of light. You can “spend” this budget entirely on moving through time, or you can spend some of it also moving through space.

The Triangle-person and the Circle-person in the comic both move at c through the spacetime, but on different paths (“worldlines”). Once separated, it will be hard for them to reunite with the exact “version” of others they left behind. Even if they meet again in space, like the Circle finding the Triangle’s “ghost,” they’ll have most likely experienced different amounts of elapsed time.

Thus, switching away from science and into philosophy, we could say that to meet the same exact person again - one with the same experience of time and space - the two characters would need to follow highly specific paths through spacetime.

Since following specific spacetime trajectories is not something that we tend to think about on a daily basis, our unique paths through the universe mean we are unlikely to ever meet the same exact person twice.